amazinggggggggg...........................

Bosco Verticale: World’s First Vertical Forest in Milan

December 19th, 2011 by The Tour Planners - Admin

A fascinating new pair of residential tower called Bosco Verticale is being constructed at Milan, Italy. Designed by architect Stefano Boeri, Bosco Verticale is being construed as “a project for metropolitan reforestation that contributes to the regeneration of the environment and urban biodiversity without the implication of expanding the city upon the territory”. Towering over the city’s skyline the world’s first forest in the sky will be a sight to behold. The 27 storied building will accommodate nearly one hectare of forest trees as tall as oak and amelanchiers in its cleverly designed balconies. The 365 and 260 foot emerald twin towers will house an astonishing 900 trees, 5,000 shrubs and 11,000 ground cover plants.

This is a concept illustration of how Bosco Verticale will look like when completed.

In summer, the trees will provide shade and filter the city’s dust; in winter, sunlight will shrine through the bare branches. Bosco Verticale’s greenery will absorb carbon dioxide and produce oxygen, while protecting the building from wind and penetrating sunlight. Boeri claims that the inclusion of trees adds just 5 percent to construction costs, and is a necessary response to the sprawl of the modern city. If the units were individual houses, it would require 50,000 sq m of land, and 10,000 sq m of woodland.

Currently, Bosco Verticale looks like this.

Important Persons of Electronics Evolution

1. In 1791 Luigi Galvani: Professor of anatomy (university of bologna) with the help of series of experiments showed the presence of electricity in animals (specifically frogs).
2. In 1799 Charles coulomb: One famous scientist of the 18th century demonstrated that there existed a force between any two charges, this force could be either attractive force or repulsive force and this force is also influenced by the distance of separation between the two charges.
3. In 1799 Allessandro Volta : An Italian scientist credited with the invention of battery. He was the first to develop a battery know as the voltaic cell that could produce electricity as a result of chemical reaction.
4. In 1820, Hans Christian oersted showed that a magnetic field is associated whenever current flows through a conductor. To demonstrate this effect he connect a copper wire to the terminals of a battery and a switch and placed a magnetic compass near the wire. When the switch was close current started flowing in the wire and a deflection was seen in the compass indicating that current carrying conductor has an associated magnetic field.
5. In 1827 George simon Ohm, a germen physicist derived the relation between the voltage applied (V), the current (I), and the resistance of a circuit. This is the famous and the most basic law Ohm’s law. It is given as V=IR.
6. In 1831 Michael Faraday , a British scientist after the discovery by Oersted that a magnetic field is associated with the conductor carrying current, Faraday through a series of experiments discovered that the vice versa is possible i.e. current can be generated by using time varying magnetic field. This phenomenon was termed as the Electromagnetic induction which is the basic underlying principle of the working of generators.
7. In 1864 James Clerk Maxwell, A British Physicist developed the electromagnetic field equations that today is referred to as Maxwell’s equations. He also formulated an important theory known as the electromagnetic theory of light, which told that electromagnetic waves travel in free space at the speed of light.
8. In 1888 Heinrich Hertz, Based on the predictions of Maxwell’s electromagnetic theory wanted to experimentally verify the theory. He performed various experiments according to what Maxwell’s theory and successfully demonstrated to the world the effect of electromagnetic radiation through space .
9. In 1895 Guglielmo Marconi put together the predictions of Maxwell and the experiments of Hertz to send electromagnetic signals through space and was successful in setting up the first transatlantic wireless communication system.
10. In 1948, William Schockley, John Bardeen and Watter Brattain developed the transistor.
11. Nikola Tesla: One great scientist without whom our today’s world would have been dark. Yes he invented alternating current (Popularly known as AC) and gave light to the world. He also has his name Tesla as the unit of magnetic field added to his credit.

Important vocabs

IMPORTANT ENGLISH VOCABS FROM – “A” (asked in exams)

Abeyance – Temporary Suspension

Abject- miserable, pitiful (दयनीय)

Abjure – To Reject

Abate – To decrease, sooth, to reduce

Accretion-   Growth by size

Accure- To Accumulate, grow by addition (इकट्ठा होना)

Astere- Unadorn, stern, Simple Person (संत)

Ambrosia- Devine Food, Delicious Food, Food of god (प्रसाद या स्वादिष्ट भोजन)

Avunculer- tolerable, be like uncle

Axiomatic- Evident without proof, Obvious

Electrical Machine
download

engineering maths

download pdf

Power System notes

Download pdf

Basic Y-Δ transformation

Δ and Y circuits with the labels which are used in this article.

The transformation is used to establish equivalence for networks with three terminals. Where three elements terminate at a common node and none are sources, the node is eliminated by transforming the impedances. For equivalence, the impedance between any pair of terminals must be the same for both networks. The equations given here are valid for complex as well as real impedances.

Equations for the transformation from Δ to Y

The general idea is to compute the impedance ${\displaystyle R_{y}}$ at a terminal node of the Y circuit with impedances ${\displaystyle R'}$, ${\displaystyle R''}$ to adjacent nodes in the Δ circuit by

${\displaystyle R_{y}={\frac {R'R''}{\sum R_{\Delta }}}}$

where ${\displaystyle R_{\Delta }}$ are all impedances in the Δ circuit. This yields the specific formulae

${\displaystyle {\begin{aligned}R_{1}&={\frac {R_{b}R_{c}}{R_{a}+R_{b}+R_{c}}}\\R_{2}&={\frac {R_{a}R_{c}}{R_{a}+R_{b}+R_{c}}}\\R_{3}&={\frac {R_{a}R_{b}}{R_{a}+R_{b}+R_{c}}}\end{aligned}}}$

Equations for the transformation from Y to Δ

The general idea is to compute an impedance ${\displaystyle R_{\Delta }}$ in the Δ circuit by

${\displaystyle R_{\Delta }={\frac {R_{P}}{R_{\mathrm {opposite} }}}}$

where ${\displaystyle R_{P}=R_{1}R_{2}+R_{2}R_{3}+R_{3}R_{1}}$ is the sum of the products of all pairs of impedances in the Y circuit and ${\displaystyle R_{\mathrm {opposite} }}$ is the impedance of the node in the Y circuit which is opposite the edge with ${\displaystyle R_{\Delta }}$. The formula for the individual edges are thus

${\displaystyle {\begin{aligned}R_{a}&={\frac {R_{1}R_{2}+R_{2}R_{3}+R_{3}R_{1}}{R_{1}}}\\R_{b}&={\frac {R_{1}R_{2}+R_{2}R_{3}+R_{3}R_{1}}{R_{2}}}\\R_{c}&={\frac {R_{1}R_{2}+R_{2}R_{3}+R_{3}R_{1}}{R_{3}}}\end{aligned}}}$

Circuit Analysis: Techniques for Solving Δ to Y

A circuit that has a combination of Δ-loads and Y-loads should be converted to the Y configuration. By converting from Δ to Y, each circuit element can be analyzed separately. Converting from Δ to Y is a technique aimed to simplify circuit analysis. (Note: harmonic behavior from the original circuit remained unchanged). The conversion from the Δ notation to Y notation is as follows:

${\displaystyle {\begin{aligned}V_{\text{LL}}={\sqrt {3}}V_{\text{LN}}\angle 30\\I_{\text{LL}}={\sqrt {3}}I_{\text{LN}}\angle -30\\Z_{\Delta }/3=Z_{\text{Y}}\\S_{3\Phi }=|S_{3\Phi }|={\sqrt {3}}V_{\text{LL}}I_{\text{L}}=3V_{\text{LN}}I_{\text{L}}\\\end{aligned}}}$

A proof of the existence and uniqueness of the transformation

The feasibility of the transformation can be shown as a consequence of the superposition theorem for electric circuits. A short proof, rather than one derived as a corollary of the more general star-mesh transform, can be given as follows. The equivalence lies in the statement that for any external voltages (${\displaystyle V_{1},V_{2}}$ and ${\displaystyle V_{3}}$) applying at the three nodes (${\displaystyle N_{1},N_{2}}$ and ${\displaystyle N_{3}}$), the corresponding currents (${\displaystyle I_{1},I_{2}}$and ${\displaystyle I_{3}}$) are exactly the same for both the Y and Δ circuit, and vice versa. In this proof, we start with given external currents at the nodes. According to the superposition theorem, the voltages can be obtained by studying the linear summation of the resulting voltages at the nodes of the following three problems applied at the three nodes with current:

(1) ${\displaystyle (I_{1}-I_{2})/3,-(I_{1}-I_{2})/3,0}$

(2) ${\displaystyle 0,(I_{2}-I_{3})/3,-(I_{2}-I_{3})/3}$ and

(3) ${\displaystyle -(I_{3}-I_{1})/3,0,(I_{3}-I_{1})/3}$

It can be readily shown by Kirchhoff's circuit laws that ${\displaystyle I_{1}+I_{2}+I_{3}=0}$. One notes that now each problem is relatively simple, since it involves only one single ideal current source. To obtain exactly the same outcome voltages at the nodes for each problem, the equivalent resistances in the two circuits must be the same, this can be easily found by using the basic rules of series and parallel circuits:

${\displaystyle R_{3}+R_{1}={\frac {(R_{c}+R_{a})R_{b}}{R_{a}+R_{b}+R_{c}}},}$ ${\displaystyle {\frac {R_{3}}{R_{1}}}={\frac {R_{a}}{R_{c}}}.}$

Though usually six equations are more than enough to express three variables (${\displaystyle R_{1},R_{2},R_{3}}$) in term of the other three variables(${\displaystyle R_{a},R_{b},R_{c}}$), here it is straightforward to show that these equations indeed lead to the above designed expressions. In fact, the superposition theorem not only establishes the relation between the values of the resistances, but also guarantees the uniqueness of such solution.

Simplification of networks

Resistive networks between two terminals can theoretically be simplified to a single equivalent resistor (more generally, the same is true of impedance). Series and parallel transforms are basic tools for doing so, but for complex networks such as the bridge illustrated here, they do not suffice.

The Y-Δ transform can be used to eliminate one node at a time and produce a network that can be further simplified, as shown.

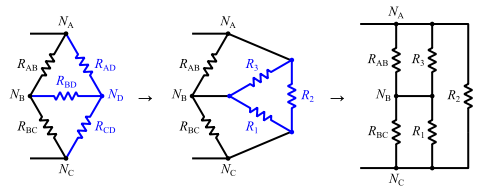
Transformation of a bridge resistor network, using the Y-Δ transform to eliminate node D, yields an equivalent network that may readily be simplified further.

The reverse transformation, Δ-Y, which adds a node, is often handy to pave the way for further simplification as well.

Transformation of a bridge resistor network, using the Δ-Y transform, also yields an equivalent network that may readily be simplified further.

Every two-terminal network represented by a planar graph can be reduced to a single equivalent resistor by a sequence of series, parallel, Y-Δ, and Δ-Y transformations.^[3] However, there are non-planar networks that cannot be simplified using these transformations, such as a regular square grid wrapped around a torus, or any member of the Petersen family.

Graph theory[edit]

In graph theory, the Y-Δ transform means replacing a Y subgraph of a graph with the equivalent Δ subgraph. The transform preserves the number of edges in a graph, but not the number of vertices or the number of cycles. Two graphs are said to be Y-Δ equivalent if one can be obtained from the other by a series of Y-Δ transforms in either direction. For example, the Petersen family is a Y-Δequivalence class.

Demonstration

Δ-load to Y-load transformation equations

Δ and Y circuits with the labels that are used in this article.

To relate ${\displaystyle \{R_{a},R_{b},R_{c}\}}$ from Δ to ${\displaystyle \{R_{1},R_{2},R_{3}\}}$ from Y, the impedance between two corresponding nodes is compared. The impedance in either configuration is determined as if one of the nodes is disconnected from the circuit.

The impedance between N₁ and N₂ with N₃ disconnected in Δ:

${\displaystyle {\begin{aligned}R_{\Delta }(N_{1},N_{2})&=R_{c}\parallel (R_{a}+R_{b})\\&={\frac {1}{{\frac {1}{R_{c}}}+{\frac {1}{R_{a}+R_{b}}}}}\\&={\frac {R_{c}(R_{a}+R_{b})}{R_{a}+R_{b}+R_{c}}}\end{aligned}}}$

To simplify, let ${\displaystyle R_{T}}$ be the sum of ${\displaystyle \{R_{a},R_{b},R_{c}\}}$.

${\displaystyle R_{T}=R_{a}+R_{b}+R_{c}}$

Thus,

${\displaystyle R_{\Delta }(N_{1},N_{2})={\frac {R_{c}(R_{a}+R_{b})}{R_{T}}}}$

The corresponding impedance between N₁ and N₂ in Y is simple:

${\displaystyle R_{Y}(N_{1},N_{2})=R_{1}+R_{2}}$

hence:

${\displaystyle R_{1}+R_{2}={\frac {R_{c}(R_{a}+R_{b})}{R_{T}}}}$   (1)

Repeating for ${\displaystyle R(N_{2},N_{3})}$:

${\displaystyle R_{2}+R_{3}={\frac {R_{a}(R_{b}+R_{c})}{R_{T}}}}$   (2)

and for ${\displaystyle R(N_{1},N_{3})}$:

${\displaystyle R_{1}+R_{3}={\frac {R_{b}(R_{a}+R_{c})}{R_{T}}}.}$   (3)

From here, the values of ${\displaystyle \{R_{1},R_{2},R_{3}\}}$ can be determined by linear combination (addition and/or subtraction).

For example, adding (1) and (3), then subtracting (2) yields

${\displaystyle R_{1}+R_{2}+R_{1}+R_{3}-R_{2}-R_{3}={\frac {R_{c}(R_{a}+R_{b})}{R_{T}}}+{\frac {R_{b}(R_{a}+R_{c})}{R_{T}}}-{\frac {R_{a}(R_{b}+R_{c})}{R_{T}}}}$

${\displaystyle 2R_{1}={\frac {2R_{b}R_{c}}{R_{T}}}}$

thus,

${\displaystyle R_{1}={\frac {R_{b}R_{c}}{R_{T}}}.}$

where ${\displaystyle R_{T}=R_{a}+R_{b}+R_{c}}$

For completeness:

${\displaystyle R_{1}={\frac {R_{b}R_{c}}{R_{T}}}}$ (4)

${\displaystyle R_{2}={\frac {R_{a}R_{c}}{R_{T}}}}$ (5)

${\displaystyle R_{3}={\frac {R_{a}R_{b}}{R_{T}}}}$ (6)

Y-load to Δ-load transformation equations

Let

${\displaystyle R_{T}=R_{a}+R_{b}+R_{c}}$.

We can write the Δ to Y equations as

${\displaystyle R_{1}={\frac {R_{b}R_{c}}{R_{T}}}}$   (1)

${\displaystyle R_{2}={\frac {R_{a}R_{c}}{R_{T}}}}$   (2)

${\displaystyle R_{3}={\frac {R_{a}R_{b}}{R_{T}}}.}$   (3)

Multiplying the pairs of equations yields

${\displaystyle R_{1}R_{2}={\frac {R_{a}R_{b}R_{c}^{2}}{R_{T}^{2}}}}$   (4)

${\displaystyle R_{1}R_{3}={\frac {R_{a}R_{b}^{2}R_{c}}{R_{T}^{2}}}}$   (5)

${\displaystyle R_{2}R_{3}={\frac {R_{a}^{2}R_{b}R_{c}}{R_{T}^{2}}}}$   (6)

and the sum of these equations is

${\displaystyle R_{1}R_{2}+R_{1}R_{3}+R_{2}R_{3}={\frac {R_{a}R_{b}R_{c}^{2}+R_{a}R_{b}^{2}R_{c}+R_{a}^{2}R_{b}R_{c}}{R_{T}^{2}}}}$   (7)

Factor ${\displaystyle R_{a}R_{b}R_{c}}$ from the right side, leaving ${\displaystyle R_{T}}$ in the numerator, canceling with an ${\displaystyle R_{T}}$ in the denominator.

${\displaystyle R_{1}R_{2}+R_{1}R_{3}+R_{2}R_{3}={\frac {(R_{a}R_{b}R_{c})(R_{a}+R_{b}+R_{c})}{R_{T}^{2}}}}$

${\displaystyle R_{1}R_{2}+R_{1}R_{3}+R_{2}R_{3}={\frac {R_{a}R_{b}R_{c}}{R_{T}}}}$ (8)

Note the similarity between (8) and {(1),(2),(3)}

Divide (8) by (1)

${\displaystyle {\frac {R_{1}R_{2}+R_{1}R_{3}+R_{2}R_{3}}{R_{1}}}={\frac {R_{a}R_{b}R_{c}}{R_{T}}}{\frac {R_{T}}{R_{b}R_{c}}},}$

${\displaystyle {\frac {R_{1}R_{2}+R_{1}R_{3}+R_{2}R_{3}}{R_{1}}}=R_{a},}$

which is the equation for ${\displaystyle R_{a}}$. Dividing (8) by (2) or (3) (expressions for ${\displaystyle R_{2}}$ or ${\displaystyle R_{3}}$) gives the remaining equations.